what happens to the foci when you flatten the orbit?
Johannes Kepler: TheLaws of Planetary Motion
In the interplay between quantitative ascertainment and theoretical construction that characterizes the evolution of modern science, we have seen that Brahe was the master of the first simply was deficient in the second. The adjacent slap-up development in the history of astronomy was the theoretical intuition of Johannes Kepler (1571-1630), a High german who went to Prague to go Brahe's assistant.
Brahe's Data and Kepler
Kepler and Brahe did non get along well. Brahe apparently mistrusted Kepler, fearing that his bright young assistant might eclipse him every bit the premiere astonomer of his solar day. He therefore let Kepler run across simply office of his voluminous information. He set Kepler the chore of understanding the orbit of the planet Mars, which was particularly troublesome. It is believed that function of the motivation for giving the Mars problem to Kepler was that it was difficult, and Brahe hoped it would occupy Kepler while Brahe worked on his theory of the Solar System. In a supreme irony, it was precisely the Martian data that allowed Kepler to codify the correct laws of planetary motion, thus eventually achieving a place in the development of astronomy far surpassing that of Brahe.
Kepler and the Elliptical Orbits
Different Brahe, Kepler believed firmly in the Copernican system. In retrospect, the reason that the orbit of Mars was particularly difficult was that Copernicus had correctly placed the Sunday at the center of the Solar Organisation, only had erred in bold the orbits of the planets to be circles. Thus, in the Copernican theory epicycles were yet required to explicate the details of planetary motion.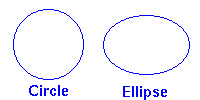 
It fell to Kepler to provide the concluding piece of the puzzle: after a long struggle, in which he tried mightily to avoid his eventual determination, Kepler was forced finally to the realization that the orbits of the planets were not the circles demanded past Aristotle and causeless implicitly by Copernicus, but were instead the "flattened circles" that geometers call ellipses (See adjacent figure; the planetary orbits are only slightly elliptical and are not as flattened equally in this example.)
The irony noted to a higher place lies in the realization that the difficulties with the Martian orbit derive precisely from the fact that the orbit of Mars was the most elliptical of the planets for which Brahe had all-encompassing data. Thus Brahe had unwittingly given Kepler the very part of his information that would permit Kepler to somewhen codify the correct theory of the Solar Organisation and thereby to banish Brahe's own theory!
Some Properties of Ellipses
Since the orbits of the planets are ellipses, permit us review a few bones properties of ellipses.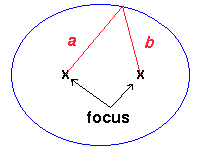 
1. For an ellipse there are two points chosen foci (atypical: focus) such that the sum of the distances to the foci from any point on the ellipse is a constant. In terms of the diagram shown to the left, with "x" marking the location of the foci, we accept the equation
a + b = constant
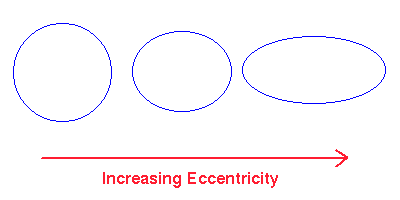
that defines the ellipse in terms of the distances a and b.2. The amount of "flattening" of the ellipse is termed the eccentricity. Thus, in the following effigy the ellipses become more eccentric from left to right. A circle may be viewed equally a special case of an ellipse with zero eccentricity, while as the ellipse becomes more flattened the eccentricity approaches one.
Mathematically information technology is defined as the distance between foci divided by the major centrality length. Thus, all ellipses have eccentricities lying between zero and one.
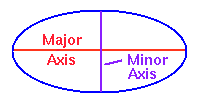3. The long centrality of the ellipse is called the major axis, while the brusque axis is called the minor centrality (adjacent figure). One-half of the major axis is termed a semimajor axis. The length of a semimajor axis is oft termed the size of the ellipse. It tin be shown that the average separation of a planet from the Sunday as it goes around its elliptical orbit is equal to the length of the semimajor axis. Thus, past the "radius" of a planet's orbit one ordinarily means the length of the semimajor axis. For a more detailed investigation of the backdrop of ellipses, encounter this ellipse applet
The Laws of Planetary Motion
Kepler obtained Brahe's data afterward his death despite the attempts by Brahe's family to go on the data from him in the promise of monetary proceeds. In that location is some bear witness that Kepler obtained the data by less than legal ways; information technology is fortunate for the development of modern astronomy that he was successful. Utilizing the voluminous and precise data of Brahe, Kepler was somewhen able to build on the realization that the orbits of the planets were ellipses to formulate his 3 Laws of Planetary Motion.Kepler's Commencement Constabulary:
| I. The orbits of the planets are ellipses, with the Sunday at 1 focus of the ellipse. |
 |
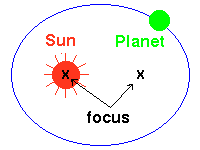Kepler'due south First Police force is illustrated in the image shown in a higher place. The Lord's day is not at the centre of the ellipse, but is instead at ane focus (generally in that location is nothing at the other focus of the ellipse). The planet then follows the ellipse in its orbit, which means that the Earth-Sun distance is constantly irresolute as the planet goes effectually its orbit. For purpose of illustration nosotros have shown the orbit as rather eccentric; remember that the actual orbits are much less eccentric than this.
Kepler's Second Police force:
| II. The line joining the planet to the Dominicus sweeps out equal areas in equal times equally the planet travels around the ellipse. |
 |
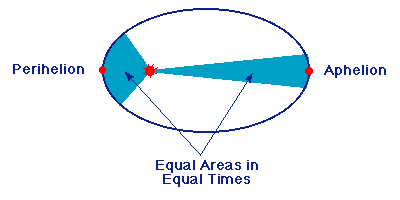Kepler's 2nd law is illustrated in the preceding effigy. The line joining the Dominicus and planet sweeps out equal areas in equal times, so the planet moves faster when it is nearer the Dominicus. Thus, a planet executes elliptical motion with constantly changing angular speed equally it moves well-nigh its orbit. The signal of nearest arroyo of the planet to the Sun is termed perihelion; the point of greatest separation is termed aphelion. Hence, by Kepler's 2d police force, the planet moves fastest when it is nigh perihelion and slowest when it is near aphelion.
Kepler's Third Law:
| III. The ratio of the squares of the revolutionary periods for two planets is equal to the ratio of the cubes of their semimajor axes: |
 |
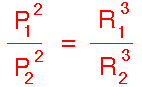In this equation P represents the period of revolution (orbit) for a planet around the sun and R represents the length of its semimajor axis. The subscripts "1" and "ii" distinguish quantities for planet 1 and 2 respectively. The periods for the two planets are assumed to be in the same time units and the lengths of the semimajor axes for the two planets are assumed to be in the same altitude units.
Kepler's Third Law implies that the period for a planet to orbit the Lord's day increases rapidly with the radius of its orbit. Thus, we find that Mercury, the innermost planet, takes simply 88 days to orbit the Sun but the outermost planet (Pluto) requires 248 years to practise the same.
Here is a java applet assuasive you to investigate Kepler's Laws, and Here is an animation illustrating the bodily relative periods of the inner planets.
Calculations Using Kepler'southward Third Law
A user-friendly unit of measurement for periods is in Earth years, and a user-friendly unit of measurement for distances is the average separation of the Earth from the Lord's day, which is termed an astronomical unit and is abbreviated as AU. If these units are used in Kepler's 3rd Law, the denominators in the preceding equation are numerically equal to unity and it may be written in the simple grade 
or for the length of the semimajor axis, given the period of the planet,
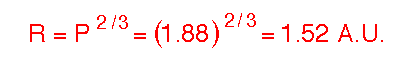
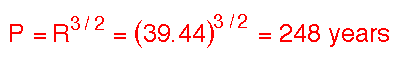
Supplementary References
- Astronomy Flick of the Day Archive
- A Short Biography of Kepler
hoinvillematurmin.blogspot.com
Source: https://www.pas.rochester.edu/~blackman/ast104/kepler11.html